Any device for converting heat energy into mechanical energy is called a
heat engine. Internal combustion engines and steam engines are both examples of heat
engines.
A heat engine takes in heat energy at a high temperature and emits some
of it at a lower temperature.
The net amount of heat flowing into an engine is equal
to the net amount of work done by the engine. This can be represented by Figure 1. An
amount of heat Q1 is taken in at the higher temperature T1 and an
amount of heat Q2 is emitted at the lower temperature T2.
The
efficiency of such a cycle is given by the equation:
Tbe concept of the ideal heat engine was first developed by the French scientist Sadi Carnot in 1824. He imagined an engine that was free from friction and where the working substance, usually a gas, was taken through a completely reversible cycle consisting of two isothermal and two adiabatic changes.
This shows that the efficiency of an ideal
heat engine depends only on the temperatures of the source and sink, and also that a heat
engine can never be 100 per cent efficient. For example at Dungeness power station steam
at 400 oC (673 K) is used to drive a turbine and is emitted to the sea at 300 K. This
gives a maximum efficiency of 55 per cent.
It would be useful for comparison
to look up the operating conditions for some of the other nuclear power stations. The greater
the operating temperature the greater the efficiency for a fixed output
temperature.
The approximate efficiencies of some practical heat engines are given
below:
| Internal combustion engine | 56% | |
| Steam engine | 40% | |
| Diesel engine | 68% | |
| Dungeness nuclear power station | 55% |
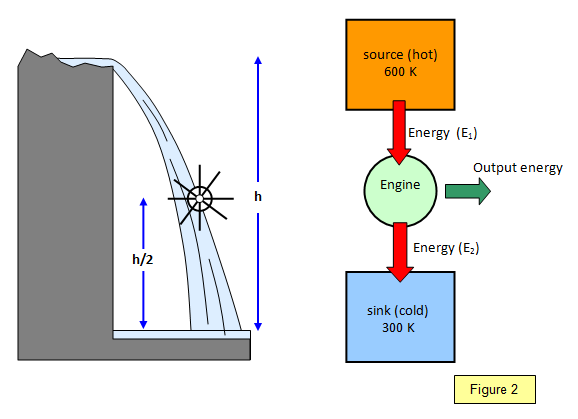
You can see how this idea works by comparing
the heat engine with a waterwheel. If the waterwheel is placed half way up the waterfall then
you can only expect to achieve half the energy output possible and so the efficiency would,
at best, be 50%. Similarly if the heat engine was operating so that the sink temperature was
half that of the source temperature then the greatest efficiency that you could hope for would
again be 50%. (see Figure 2)
Note that this is the maximum possible – in practice
the actual efficiency would be smaller.